What is Method of Joints? 5 Steps of Method of Joints

The method of joints is a procedure used to solve for the unspecified forces acting on members of a truss and to solve for all the unknown forces in a truss structure, the method of joints is usually the fastest and easiest way.

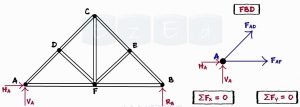
For determining the force acting on the individual members of a truss the method of joints is one of the simplest methods because it only requires two force equilibrium equations.

Using the Method of Joints:

The process used in the method of joints is given below;

• It is usually useful to label the members and the joints in your truss in the beginning and this will help you keep everything organized and compatible in later analysis.

• As a rigid body, treat the entire truss structure then draw a free body diagram and write out the equilibrium equations. Then for the external reacting forces acting on the truss structure solve the equations and this inspection should not differ from the inspection of a single rigid body.

• Let suppose that there is a pin or some other small amount of material at each of the connection points between the members and for each connection point, draw a free body diagram. Remember to include;

1. Any load forces or any external reaction that may be acting at that joint.
2. To that joint, a normal force for each two force member is connected and always know that between the two connection points on the member for a two force member, the force will be acting along the line. We also need to estimate if it will be a tensile or a compressive force and incorrect estimate lead to a negative solution.
3. Any positive forces will be tensile forces and any negative forces will be compressive forces later in the solution.
4. In the diagram, mark each force and involve any known magnitudes and directions and for each unknown, deliver variable names.

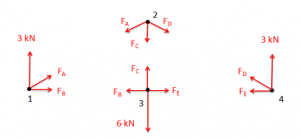
• For each of the joints, write out the equilibrium equations so, there will be force equations but no moment equations and this should give you a large number of equations with either two as for 2D problems or three as for 3D problems equations for each joint.

The sum of the forces in the x-direction will be zero and the sum of the forces in the y-direction will be zero for each of the joints in planar trusses.

The sum of the forces in the x-direction will be zero, the sum of the forces in the y-direction will be zero, and the sum of forces in the z-direction will be zero for each of the joints in space trusses.

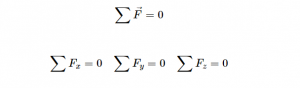
• Finally, for the unknowns, solve the equilibrium equations and for everything, at once, you can use matrix equations to solve. Remember that in the member’s negative answers indicate compressive forces.